1376. Time Needed to Inform All Employees
A company has n employees with a unique ID for each employee from 0 to n - 1. The head of the company has is the one with headID.
Each employee has one direct manager given in the manager array where manager[i] is the direct manager of the i-th employee, manager[headID] = -1. Also it’s guaranteed that the subordination relationships have a tree structure.
The head of the company wants to inform all the employees of the company of an urgent piece of news. He will inform his direct subordinates and they will inform their subordinates and so on until all employees know about the urgent news.
The i-th employee needs informTime[i] minutes to inform all of his direct subordinates (i.e After informTime[i] minutes, all his direct subordinates can start spreading the news).
Return the number of minutes needed to inform all the employees about the urgent news.
Example 1:
Input: n = 1, headID = 0, manager = [-1], informTime = [0] Output: 0 Explanation: The head of the company is the only employee in the company.
Example 2:
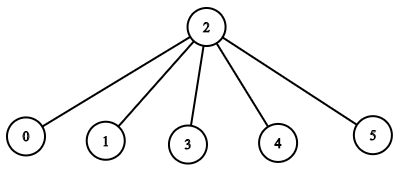
Input: n = 6, headID = 2, manager = [2,2,-1,2,2,2], informTime = [0,0,1,0,0,0] Output: 1 Explanation: The head of the company with id = 2 is the direct manager of all the employees in the company and needs 1 minute to inform them all. The tree structure of the employees in the company is shown.
Example 3:
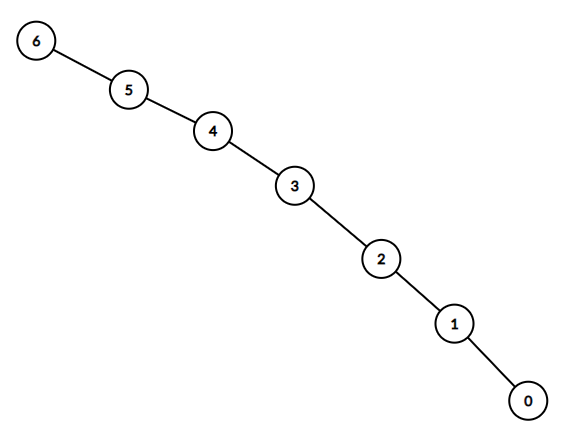
Input: n = 7, headID = 6, manager = [1,2,3,4,5,6,-1], informTime = [0,6,5,4,3,2,1] Output: 21 Explanation: The head has id = 6. He will inform employee with id = 5 in 1 minute. The employee with id = 5 will inform the employee with id = 4 in 2 minutes. The employee with id = 4 will inform the employee with id = 3 in 3 minutes. The employee with id = 3 will inform the employee with id = 2 in 4 minutes. The employee with id = 2 will inform the employee with id = 1 in 5 minutes. The employee with id = 1 will inform the employee with id = 0 in 6 minutes. Needed time = 1 + 2 + 3 + 4 + 5 + 6 = 21.
Example 4:
Input: n = 15, headID = 0, manager = [-1,0,0,1,1,2,2,3,3,4,4,5,5,6,6], informTime = [1,1,1,1,1,1,1,0,0,0,0,0,0,0,0] Output: 3 Explanation: The first minute the head will inform employees 1 and 2. The second minute they will inform employees 3, 4, 5 and 6. The third minute they will inform the rest of employees.
Example 5:
Input: n = 4, headID = 2, manager = [3,3,-1,2], informTime = [0,0,162,914] Output: 1076
Constraints:
1 <= n <= 10^50 <= headID < nmanager.length == n0 <= manager[i] < nmanager[headID] == -1informTime.length == n0 <= informTime[i] <= 1000informTime[i] == 0if employeeihas no subordinates.- It is guaranteed that all the employees can be informed.
一个公司有n个工人,每个工人有一个直属上级,公司最大的老板没有直属上级。当老板要下发通知时,他会通知他的直属下级,直属下级又一级一级往下通知。每个上级通知其所有下级的用时不同。问老板的通知要过多久能传达给所有工人。
这是一题多叉树的问题。本质上是求从根节点到所有叶子节点中的最长路径是多少。
想到树的问题一定要想到递归解法。对于任意一棵子树,【它到所有叶子节点的最长路径】等于【它到所有直属下级的长度】加上【所有直属下级到它们各自的所有叶子节点的最长路径的最大值】之和。
完整代码如下:
class Solution {
public:
int maxTime(int headID, map<int, vector<int>>& employees, vector<int>& informTime) {
if (employees.find(headID) == employees.end())return 0;
int cur_time = informTime[headID];
int child_max = 0;
for (int i = 0; i < employees[headID].size(); ++i) {
int cur_child = maxTime(employees[headID][i], employees, informTime);
child_max = max(child_max, cur_child);
}
return cur_time + child_max;
}
int numOfMinutes(int n, int headID, vector<int>& manager, vector<int>& informTime) {
if (n == 1)return informTime[0];
map<int, vector<int>> employees;
for (int i = 0; i < n; ++i) {
employees[manager[i]].push_back(i);
}
return maxTime(headID, employees, informTime);
}
};本代码提交AC,用时1548MS。